I mentioned that I find myself working Sudoku puzzles lately. My other spare-time hobby, relentless nerd that I am, is analyzing the 12-tone pieces I’m using for my 12-tone analysis class in the fall. The two activities – tone-row searching and Sudoku – are kind of alarmingly similar, so much so that I can forget at times which I’m doing. (Is that “aggregate” filled up yet? Am I looking for 12 of something, or 9 of something?) I do like understanding things, though, so that I get a real childlike kick out of teasing out the structure of a piece I’ve been listening to for decades. In other words it’s more like summer fun for me than it would be, I imagine, for most people.
I’m also honing in on the repertoire for the course. Looking for Schoenberg 12-tone pieces I can stomach, I’ve come up with the Waltz, Op. 23 No. 5, the first 12-tone piece he published, with a row that never transposes; the Op. 24 Serenade sonnet with the 11-syllable lines that go out of phase with the row; and the magnificent first scene of Moses und Aron. For Webern I am almost criterion-less, because they all make the same point, and I already use my favorites – Opp. 21, 27, and 29 – in other courses. Using the Concerto feels like such a cliché, but I guess my students need to know the clichés. I’m afraid I’m at the point of dropping poor Aaron Copland from the list. Connotations and Inscape are big, unwieldy pieces, and I just don’t think they’re that good, and I don’t want to end up weakly defending them. Copland’s imagination seemed constrained by the technique. If I’m going to venture into a large orchestral work (in addition to Sinfonia), I’d much rather use Rochberg’s Second Symphony, which is the most exciting, memorable, and followable orchestral 12-tone work by any American I know of – also more to my taste, frankly, than anything the Second Vienna School ever produced. I’m already using Rochberg’s Serenata d’Estate, which has been fun to take apart.
I have to do much of this at the beginning of the summer, because I need to order scores for students, and I have to make sure I don’t get in over my head. I don’t want to omit Boulez, and I’ll attempt Le Marteau rather than more attractive examples only because there’s a published analysis. Yes, I’m slowly working my way through Lev Koblyakov’s oddly titled Pierre Boulez: A World of Harmony, a stunning work of analysis, and an equally stunning piece of dismal writing. If he could have stuck with even one musical passage long enough to show how Boulez derived it from beginning to end, it would be immensely more illuminating, but instead he goes concept by concept and jumps all over the piece with each new concept. He’s certainly concise – too much so, in fact – and I admire his achievement, but he could have made his findings infinitely easier to digest.
(Time magazine hasn’t yet added it to their online archive, but I clearly remember around 1980 when they ran an article breaking the news that a music-analyst, Koblyakov, had cracked the code to Le marteau. Amazing to think that mainstream media actually cared about such things a mere 30 years ago.)
Nevertheless, I get that Boulez divides up his row into five segments in five possible ways based on a rotating number series:
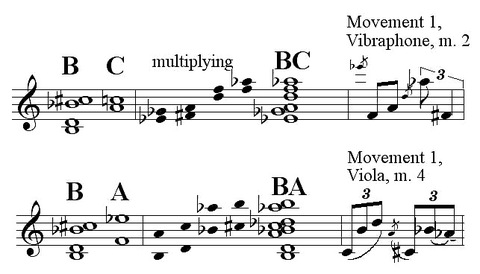
and so on. I also get that he “multiplies” each of those five segments by all five of them to build up derived unordered pitch sets – the process of “chord multiplication” being to transpose one chord to all the pitches of the other chord and add all the pitches together. And you can see how (if you take the trouble) each gesture is drawn from the pitches of these chord-multiplication products:
I also get how Boulez chose the order of chord multiples by making little diagonal patterns through his chart of available sets. Sounds like fun.
Well, that’s great, sir, you’re a Lebowski, I’m a Lebowski. What I can’t see is why this method of generating pitches has any significant advantage over Cage’s chance processes, which Boulez so vehemently rejected. I can’t see what they have to do with the ostensive unifying purpose of the 12-tone row, and since Boulez plays around within them as unordered collections, plus has two of them going at any given time in extremely rapid succession (any one collection rarely occupying more than two beats at quarter = 168), I can’t see what purpose this incredibly convoluted process serves in the least. Stephen Heinemann in “Pitch-Class Set Multiplication in Theory and Practice” (Music Theory Spectrum Vol. 20, No. 1, Spring 1998) promises to reveal a “process-based listening strategy” for Le Marteau based on all this, but by the time one’s waded through all his math, the results aren’t much. He shows how in “Domain 5” (one of the five harmonic areas) a certain octatonic partitioning tends to occur, but then writes
the other domains do not parse as easily as Domain 5, and… such an analytical approach is not without its obstacles. The aural “processing” in terms of interval class 3 and octatonic structuring is complicated… by the sheer rapidity of change…
If this is the best assurance we can get from someone who understands Le Marteau well enough to correct Koblyakov’s misconceptions about it, I’m ready to give up on anyone ever making detailed aural sense of the piece. As Fred Lerdahl famously wrote (and Taruskin quotes it in his history),<
Boulez’s Le Marteau sans Maitre (1954) was widely hailed as a masterpiece of post-war serialism. Yet nobody could figure out, much less hear, how the piece was serial. From hints in Boulez (1963), Koblyakov (1977) at last determined that it was indeed serial, though in an idiosyncratic way. In the interim, listeners made what sense they could of the piece in ways unrelated to its construction. Nor has Koblyakov’s decipherment subsequently changed how the piece is heard…. The serial organization of Le Marteau> would appear, 30 years later, to be irrelevant. The story is, or should be, disturbing. (“Cognitive Constraints on Compositional Systems,” in Generative Processes in Music, Oxford, 1988)
I agree. I’m disturbed by it. And yet…
* * * * * * * * * * * * * * * * * * * * * *
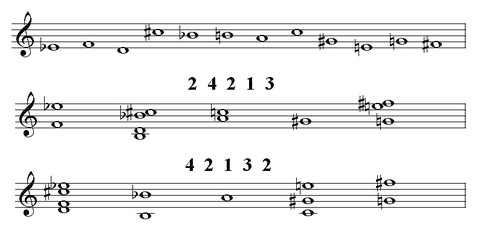
– and yet, just as I couldn’t decipher Le Marteau on my own without Koblyakov’s almost grudging help, neither had I been able to tease any detailed sense out of Stravinsky’s Requiem Canticles before Joseph Straus’s Stravinsky’s Late Music appeared. (Koblyakov proudly asserts that he analyzed Le Marteau without seeing Boulez’s sketches, which are apparently lost, but I gather Straus had access to Stravinsky’s notes.) In my youth I struggled vainly to relate the luscious quadruple flute chords from the Interlude (so proto-Feldmanesque, although Feldman was already doing similar things) to any kind of pitch order drawn from the first movement:
What a relief it was to learn that the chords are drawn from the first two-note columns in an array of four rows, prime, retrograde, inversion, and retrograde inversion:
And, going on, the two chords in the fifth measure are drawn from columns 3 and 4, respectively. The relationship of this fat A-flat 9 chord, drawn from two notes each from four different row forms, with duplications, to any sense of this work’s 12-tone content is just as tenuous as the order of Boulez’s chord multiplications. Here and elsewhere, it’s almost like Stravinsky wrote out his row forms in some interesting logical order and then just glanced around for groups of pitches he liked that happened to be adjacent on the page. Of course, that gorgeous chord locked in the choice of other chords following it, quasi-randomly determined, with little musical function of their own but to resolve back to the initial one. The approach seems more charmingly intuitive, almost accidental or even opportunistic, than theoretical. I’m reminded of Bill Duckworth’s piece from the 1970s Pitch City, in which the players are given a map for improvising their way through a row matrix.
(I was also amused to read in Straus’s book about Boulez’s and Stravinsky’s relationship. Despite the flattering interest Stravinsky showed in the young Boulez, Boulez led a disastrous 1958 performance of Stravinsky’s Threni, and frequently made public his contempt for all of Stravinsky’s post-1923 music. In 1970 Stravinsky said, “I have not had any contact with M. Boulez myself since, shortly after visiting me in Hollywood three years ago, he talked about my latest compositions… with unforgivable condescension, then went on to play them at a prestigious concert in Edinburgh. This was not the first proof of disingenuousness I had had of that arch-careerist, but it will be the last in which I have any personal connection.” (Stravinsky’s Late Music, p. 34, n. 66) I’m not sure “arch-careerist” is the precise term for someone who bites the hand that could feed him, but I do feel certain that, even with my stunted sense of political advantage-taking, I would have returned the solicitations of Igor Stravinsky more graciously than that.)
In neither the case of Le Marteau nor Requiem Canticles does the technique seem to have any perceptible relation to the unifying idea of a 12-tone row. In the case of Requiem Canticles, though, it doesn’t matter to me, because I’ve always loved the piece and I always will; it and Threni are among my favorite Stravinsky works, which I guess makes me a pretty rare breed of Stravinsky fan. (Straus goes concept-by-concept too, but with his broad hints I’ve been able to trace the rows through half of Threni.) I’m curious to find out how Requiem Canticles was composed, but the knowledge won’t influence the way I listen to it, and I didn’t care what the process “turned out” to be. In Le Marteau‘s case, however, I have always been baffled by the music, was never able to learn to love it or even like it or remember any of it, and finding out what total disregard for perceptibility it was written with is more likely to reinforce my dismissive attitude toward it than to make me listen more sympathetically. (I’m with this guy.) And yet I do love some of Boulez’s later music, particularly Pli selon pli and Rituel.
Going further into all this is bracing me for the big philosophical question I’m expecting from the students this fall: Why use 12-tone method? What was the point? I don’t expect to have an answer by then. Already when I mentioned in modernism class that some composers deviate from strict use of the row, they became indignant; if you believe in the theory, they said, you should trust it devoutly, and if not you should abandon it. (Young people can afford to be so pure.) The music itself will have to convince them that it’s not so black and white: that the row is sometimes a unifying factor, sometimes a melody, sometimes a note generator, sometimes a pretext, sometimes an ideological weapon, sometimes a bad idea entertained for too long, sometimes even a Rorschach test. But I think I can convince them some beautiful music happened in spite of it, if not always because of it.