To teach undergraduate music theory is to recount over and over and over, year after year without variation, facts, terminology, and principles that haven’t changed since well before I was born. But Wednesday I managed to teach something new, and got a real kick out of it: Neo-Riemannian theory (named for the German musicologist Hugo Riemann, 1849-1919). I had never heard of the subject until the 2007 Minimalism conference in Wales, where Scott Alexander Cook applied the methodology to the music of Gavin Bryars (PDF). The idea is that relationships between triads can be characterized by variously close or distant pitch replacements, categorized by the three functions P (parallel), L (leading tone), and R (relative). The P function moves the third of a triad to change it from major to minor, or vice versa:
The L function lowers the root of a major triad a half-step, or raises the fifth of a minor triad a half-step, creating a new triad of the opposite modality in either case. The R function raises the fifth of a major triad a whole-step to produce the triad of the relative minor, or lowers the root of a minor triad a whole-step. And there are some other derived functions, such as D, which does from a triad to its dominant, or vice versa. And functions can be combined, so you get PL, RP, LPL, and so on. If I’m oversimplifying or getting it wrong, some kind reader will correct me.
The occasion of my bringing this up was my biennial analysis of a gorgeous passage from Liszt’s Années de Pelerinage, the beginning of “Sposalizio,” which is hardly complicated, but notoriously recalcitrant to Roman numeral analysis:
Now instead of calling that i-VI in E minor and wondering where in hell the B-flat major came from, I could label the sequence L, RLRL (or DD, since C to B-flat is two dominant jumps), PR, D, PR. It doesn’t really explain the music – though the PR does make clear the equivalent jumps from B-flat to D-flat and A-flat to B, which the enharmonic pitch notation hides – it just gives me a way to label Liszt’s key jumps, more radical in their unconcern for tonality than Chopin’s or Schumann’s. And I think the students enjoyed learning a theoretical technique that wasn’t from the musty, immemorial past, but evolved during their lifetimes.
The even greater interest for me is the potential application to my own music. In 1983, I switched over, with some trepidation, to writing in a triadic style, though not at all functionally tonal. I had been studying Bruckner and taking tips from passages like this wonderful one from his Eighth Symphony:
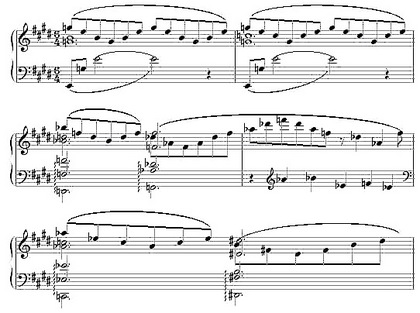
(Interestingly, in the immediate repeat of this passage Bruckner replaces the RP with a PR, and ends up in D major instead of A-flat.) I soon found myself rather obsessed with what I now learn are called LPL and RPR transformations (the latter yielding a tritone transposition). Here’s Baptism, from 1983:
The chord changes, if I have parsed them correctly with my amateur notions of Neo-Riemannian terminology, are PLP, RPR, LPL, RPR, LPL, LPL, RPRP, LPL. Note that no chord change requires fewer than three transformations – that was my conscious discipline for the piece, but of course I wasn’t thinking of it in Neo-Riemannian terms, which hadn’t been invented yet. This was pretty much my default harmonic language from Baptism of 1983 to my “Last Chance” Sonata of 1999. In 2000 I studied jazz harmony with John Esposito, and switched over to bebop chords. My microtonal music uses a process of micro-interval voice-leading that Harry Partch called “Tonality Flux,” related to Neo-Riemannian transformations, but of course not reducible to them. However, the large-scale tonal structure of my 2002 microtonal chamber opera Cinderella’s Bad Magic consists of a chain of simple Riemannian pitch shifts, all tuned to pure triads and evolving from E-flat major to C double-sharp minor, through single note-shifts R P R P L P R P L:Â
These Neo-Riemannian labels neither explain nor justify my music, but they do give me simple ways to refer to my voice-leading methods as classes of harmonic transforms. And, as papers at the last minimalism conference proved, Gavin Bryars and John Adams were using a similar kind of non-functional harmonic consistency from the early 1980s on as well. This terminology can make it easier to point out how the harmonic practices of Liszt, Bruckner, and Reger returned, following the period of widespread atonality, to form a new common practice in minimalist and especially postminimalist music – starting, I think we’d have to say, with Einstein on the Beach, whose “Spaceship” scene may succumb to only this kind of analysis. Neo-Riemannian theory may fill in some cracks in our analysis of Romantic music, when dealing with composers who couldn’t abide within the Germanic idea of a centralized tonality. For postminimalism, it may prove to be the analytical technique that fits the music like a glove.